QS 381 Winter 2002. Exam 1. January 18,2002 Time: 50 minutes. Put name on top of paper.
(1) During a run of sockeye salmon in lake Washington, a study was made
of the number of fish passing through the Chittenden Locks fish
ladder. One objective of this study was to determine the percentage of days
that more than 425 fish used the ladder. Suppose the mean for the data
was 375 fish per day and the standard deviation was 30 fish.
(a) (8 pts)What can you say about the percentage of days that more than 425
fish used the ladder?
(1) (a) at most 36% ,
k=(425-375)/30 = 1.67, 1-1/k^2 = 0.64, 1-0.64=0.36
(b) (8 pts)What is your answer to part (a) if you know that the relative
frequency distribution for the data is bell-shaped and the standard deviation
is 25 fish?
(b) around 2.5%, where 95% of the
data fall within 2 SD’s, 1-0.95 = 5/2 = 0.025
(2) Use the information from the following data set to complete the table.(7
pts)
|
Measurement Class |
Relative
Frequency |
Frequency |
Cumulative
Frequency |
|
0 <= x < 2 |
0.10 |
20 |
20 |
|
2 <= x < 4 |
0.15 |
30 |
50 |
|
4 <= x < 6 |
0.05 |
10 |
60 |
|
6 <= x < 8 |
0.45 |
90 |
150 |
|
8 <= x < 10 |
0.25 |
50 |
200 |
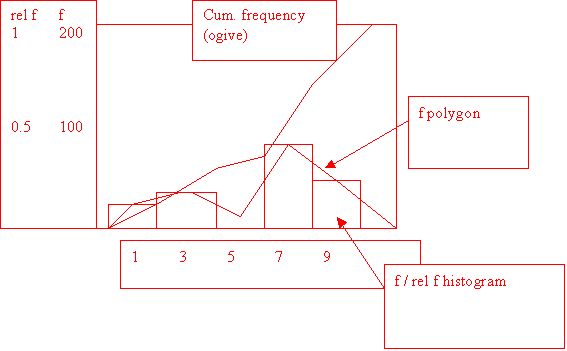
![]() Draw
the following for these data:
Draw
the following for these data:
(a)(5 pts) frequency histogram
(b)(5 pts) frequency polygon
(c) (5 pts) relative frequency histogram
(d) (5 pts) frequency ogive
(e) (5 pts) Compute the sample mean
Sum(x*f)/Sum(f) where x = midpoint
(1*20+3*30+5*10+7*90 + 9*50)/200 = (20+90+50+630+450)/200
= 1240/200 = 6.2
(3) Define the kind of sampling and the type of data for each of the following. Give a reason for your answer.
(a) (4 pts) The density of E. coli in 1cc samples of saliva from 40 male and
25 female patients in Harborview medical center.
stratified sampling, with two strata, male and female; ratio
data
(b) (4 pts) The size of fish caught by 120 anglers sampled through
binoculars from by an observer at Matthews Beach on Lake Washington.
convenience sampling (only those angler's visible from that
point) could also argue random
sample is ordinal, could be in categories small, medium or large, rankable but
no intervals
(c) (4 pts) The diameter at breast height (DBH) of 20 tree samples
apiece taken from each of 3 chosen stands at Pack Forest,
Washington (Note: there are more than 3 stands at Pack Forest).
Cluster sampling, because only
some stands chosen. ratio data (true 0)
(d) (4 pts) The barometric pressure taken every quarter hour over a 10 day
period in May on the UW Campus.
Systematic sample, interval
measure. I'm not sure if it is ratio. Accept either answer.
***If you wrote interval and only got partial credit, return your exam to Maureen for credit****
(4) Wildlife biologists trapped field mice in a particular field using an
experimental trap. They were interested in how long it would take the mice to
escape from the trap. Ten mice captures were observed. Their times to
escape (in minutes) are recorded below. The N's represent two rats that had
still not escaped after 3 hours. The data are:
100, 38 , N , 122 , 95 , 116 , 56 , 135 , 104 , N
(a) (6 pts) Can the mean be calculated for this data set? If the answer is yes,
report the value. If the answer is no, explain why not.
(4) (a) No, you need all
the values of all the data points to calculate the mean
(b) (6 pts) Can the median be calculated for this data set? If the answer is
yes, report the value. If the answer is no, explain why not.
The data can be ordered, and the median taken from the ordered data:
 38 56 95 100 104 116 122 135 N N
38 56 95 100 104 116 122 135 N N
(5) A researcher is interested in the variability in leaf size (in cm) on a
particular bigleaf maple tree early in the spring. He collected the following
10 data points, shown in the stem and leaf diagram. Leaf units are
in tenths. Note there are more than one kind of leaf in this problem. For these
data find:
1 | 1 = 1.1
|
Stem |
Leaf |
|
0 |
3 1 5 |
|
1 |
1 0 |
|
2 |
2 1 2 |
|
3 |
1 8 |
(a)(4 pts) the mean
(5) a) mean = 1.64
(b) (4 pts) the mode
b) mode = 2.2
(c) (4 pts) the range
c) range = 3.7
(d) (4 pts) the median
d) median=1.6
 (e)
(8 pts) Draw a box and whisker diagram for these data above the following line:
(e)
(8 pts) Draw a box and whisker diagram for these data above the following line:
e) maximum =3.8, min=0.1,
Q1=0.5,Q3=2.2
___________________________________________________________________________________
0
1
2
3
4