QS381A Exam 2. February 1, 2002. Please write your name on the top of the paper.

1. Scientists from universities in the United States were surveyed recently to determine readership of journals. Fifty percent of the scientists read Nature, 60 percent read Science and 20 percent read both.

a) (5 pts) Find the probability that a university scientist selected at random reads either Science or Nature or both.

0.60+0.50-0.20 = 0.90

b) (5 pts) What is the probability the scientist reads neither Science nor Nature?

1-0.90 = 0.10

2. (15 pts) Two cards are drawn from a deck of 52 playing cards (without replacement). What is the probability they are both the same suit?

4(13/52*12/51) = 52/52*12/51 = 0.235

OR

4(₁₃C₂/₅₂C₂) = 0.235

Since there are four suits, you must count the individual probability four times, because this can happen to any of the suits

3. The following table shows the joint probability (relative frequency) distribution for the type and size of hospital in a particular region.

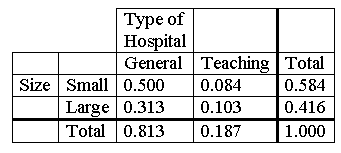
If a hospital in the region is chosen at random what is the probability that it is:

(a) (4 pts) a teaching hospital

0.187

(b) (4 pts) a large teaching hospital

0.103

(c) (4 pts) a large hospital, given that it is a teaching hospital

0.103/0.187 = 0.551

(d) (4 pts) a teaching hospital, given that it is a large hospital.

0.103/0.416 = 0.248

4. According to government data, 20% of American children under the age of 6 live in households with incomes less than the official poverty level. A random sample of 15 children under the age of 6 is selected for a study of learning in early childhood.

(a) (8 pts) Find the probability that exactly 3 children in the sample come from poverty-level households.

bin(15, 0.20), P(X=3) = ₁₅C₃ (0.20³) (0.80)¹² = 0.250

(b) (6 pts) What is the mean number of children in such a sample who come from poverty-level households? What is the standard deviation of this number between repeated samples?

E(X) = np = 15*0.20 = 3.0

variance=npq=2.4

SD = SQRT(Var) = 1.549

5. In a study of the Tucumara Indians on the upper Amazon, Tintin discovers that, on average, 5 per year are killed by digger wasp bites. Assuming that digger wasp mortality follows a Poisson distribution find the probability that

a) (8 pts) at least 3 Tucumaras per year are killed by digger wasps

P(X≥3) = 1-P(X<3) = 1-[P(X=0,1,2)]

= 1-0.125 = 0.875

b) (8 pts) more than 4 are killed a year by digger wasp bites

P(X>4) = 1-P(X≤4) = 1-[P(X<3)+P(X=3,4)]

= 1-(0.125+0.14+0.175)

= 1-0.44 = 0.56

c) (8 pts) How do these numbers compare to the same statistics for the neighboring Aracahua tribe where, on average, 8 persons per year are killed by digger wasp bites?

mu = 8, P(X≥3) = 0.986

P(X>4) = 0.90

With a higher average, these probabilities are greater

6. Makah Indian biologists are observing the northward migration of Gray Whales from Cape Alava, Washington. The following table gives the probability distribution of numbers of whales observed per hour

Number	0	1	2	3	4	5	>5
probability	0	0.24	0.32	0.18	0.16	0.07	x

a. (5 pts) What is the value of x?

1-0.97 = 0.03

b. (5 pts) What is the probability of observing fewer than 3 whales per hour?

0+0.24+0.32 = 0.56

c. (5 pts) What is the probability of observing between 2 and 4 whales per hour (inclusive)?

0.32+0.18+0.16 = 0.66

d. (5 pts) What is the probability of observing at least 4 whales per hour?

0.16+0.07+0.03 = 0.26

e. (extra credit – 10 pts) Assuming the number observed per hour in any hour is independent of the previous hour’s observation, and that the Makah will launch whaling boats when they observe 5 or more whales in an hour, what is the probability that they will have to wait 4 or more hours before launching?

A “success” is observing ≥5 whales in an hour, P(“S”) = 0.03+0.07=0.10

P(X≥4) where X~geometric(0.10), X = 1,2,…

1-P(X<4) = 1-[P(X=1,2,3)]

P(1) = pq⁰ = .1

P(2) = pq = .1(.9) = .09

P(3) = pq²= .1(.81) = .081

P(>=4) 1- P(1) – P(2) – P(3) = 0.729