Homework 6 Solutions
Page 259, #6
zc = 1.44
Page 259, # 12
![]()
Page 260, #20
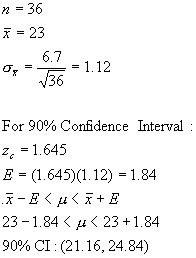

![]()
Page 260, #26

Page 261, #34
![]()
Page 262, #38
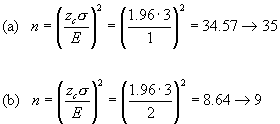
Compare
results: When you increase the amount
of acceptable error (E), the sample size required is smaller.
Page 271, #8
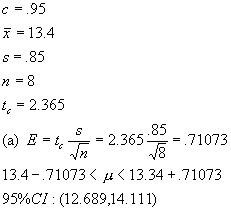
(b)
If the Normal distribution is used, zc = 1.96, and the 95%
confidence interval for m is (12.811, 13.989). So, the t
distribution gives a wider interval.
Page 272, #16
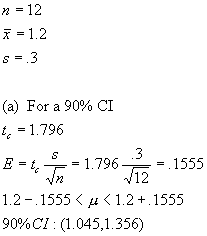
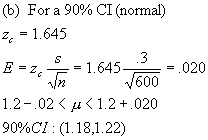
Page 273, #22

Page 273, #24

Page 273, #28
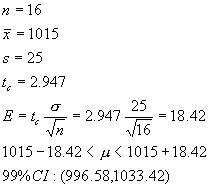
Since
1000 hours falls within the confidence interval, it can be concluded that they
are making good lightbulbs.
Page 280, #14
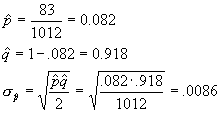
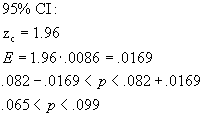
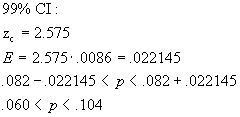
The 99% confidence interval is wider.
Page 281, #20
(a) 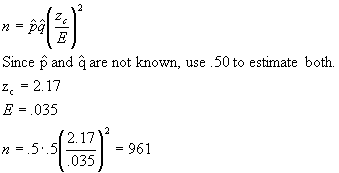
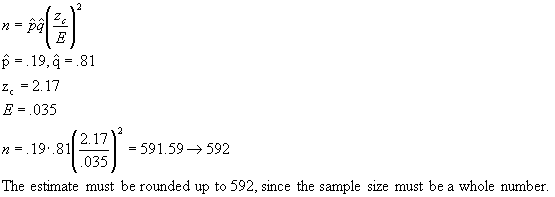 (b)
(b)
(c) If you have an estimate for the proportion (that is not 0.5), the sample size needed will always be smaller. Information about the proportion helps to reduce sample size.
Page 288 #8
(.00069,.0022) (.0247,.04690)![]()
![]()
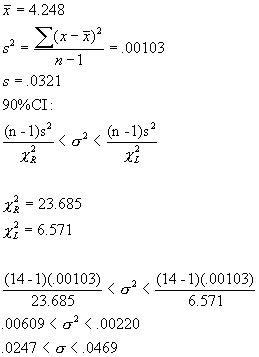
Page 289 #14

Page 289 #18
Not really acceptable, since the criteria calls for s < .025 and the lower limit of the CI ~
.025 (rounding up .0247), meaning within 90% confidence limits the std deviation
is too high.