Introduction to Geographic Information Systems in Forest Resources
| Introduction to Geographic Information Systems in Forest Resources |
|
|||||||||||||||
|
|||||||||||||||
Navigational methods based on landmarks and celestial objects has also been the standard for finding and mapping one's way.
The Global Positioning System (GPS) has revolutionized both surveying and navigation. GPS is one of the single largest breakthroughs in GIS since its inception. GPS brings the use of a locational tool, whose accuracy and precision rivals most surveying systems in use up to the present. Furthermore, the GPSs ease of use makes it an attractive technological answer to locational and surveying needs of everyone from hunters and hikers to scientists and licensed surveyors.
The ability of most GPS receivers to gather locational and attribute data endows the technology with a central place in the building new datasets as well as the verification of existing spatial datasets.
For a more complete and detailed treatment of GPS, see the Trimble Navigation homepage, or Global Positioning System Overview at the U of Colorado at Boulder.
History of the GPS Ground Control Stations Satellites Measurements of distance Precision timing Satellite location Dilution of precision Differential correction Attribute recording Import into the GIS GPS Demonstration
GPS traces its modern roots back to radio-wave locational technologies such as the LORAN system developed during WWII. A continuous development of radionavigation has progressed into the current NAVSTAR and GLONASS space-borne satellite-based navigation systems in use today. For a more full treatment of the history of GPS, see the Historical Timeline of Radionavigation Systems at the Red Sword Corporation's Home Page.
The current GPS was developed during the latter part of the Cold War, so that nuclear-equipped submarines could quickly determine their location upon surfacing, so as to calculate a rocket trajectory for ICBM targeting. In addition to this, GPS was developed for accurate position and navigation for ground forces. Because the initial applications for the system was military/defense, the system has been, and still is, operated by the Department of Defense.
Several ground control stations exist for the GPS. The Master Control facility is located at Schriever Air Force Base (formerly Falcon AFB) in Colorado. The control stations use precise RADAR equipment to determine each satellite's location, altitude, and velocity. The monitor stations also measure signals from the satellites which are incorporated into orbital models for each satellites. The models compute precise orbital data (ephemeris) and clock corrections for each satellite. The master ground station transmits these corrections for the satellite's ephemeris constants and clock offsets back to the satellites themselves. The satellites can then incorporate these updates in the signals they send to GPS receivers.
The other stations are located at Air Force stations in Hawaii (eastern Pacific Ocean), Diego Garcia (in the Indian Ocean), Kwajalein Atoll (western Pacific Ocean), and Ascension Island (central Atlantic Ocean).
The ground control section of the GPS is extremely important; without accurate and precise ground control, the satellites themselves would send incorrect data back to the GPS receivers, which would result in incorrect location calculations.
The satellites used for GPS are owned by the taxpayers of the USA and operated by the US Department of Defense (DOD). A full complement of 24 satellites is used for the system. As older satellites age, they are replaced by newer satellites.
The satellites themselves orbit the earth once every 12 hours at about 20,000 km altitude. Each satellite sends out a stream of data, converted to microwave signals, consisting of several types of information. These include such data as
The full constellation of satellites, ground control, and other infrastructure represents billions of dollars of investment. Each satellite alone costs hundreds of thousands of dollars. Remember, you paid for it, and it is your right to use it!
GPS works by solving a series of simultaneous trigonometric and algebraic equations based on angles and distances. As we know,
distance = rate * time
The microwave signals from GPS satellites are electromagnetic radiation, which we know travels at the constant rate of 299,792,458 m/s (180,000 mi/s).
The GPS signals contain the precise time of transmission, and GPS receivers contain extremely accurate clocks. Thus, it is possible to measure the time of travel for each signal. With rate and time, we can easily solve for distance.
So for each GPS signal, we can determine our distance from the satellite. However, this poses a problem, as illustrated in this image:
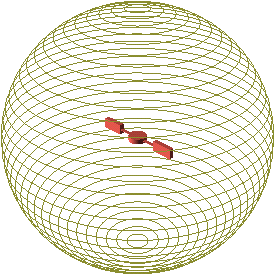One satellite signal
If we receive a single satellite signal, we would be able to determine how far away we are from the satellite, but we have no way of knowing in which direction. A single satellite signal places us on the surface of a sphere, at a given distance from the satellite. The location could be on earth, or far out in space.
If we have a second satellite, then we can place ourselves on the intersection of two spheres.
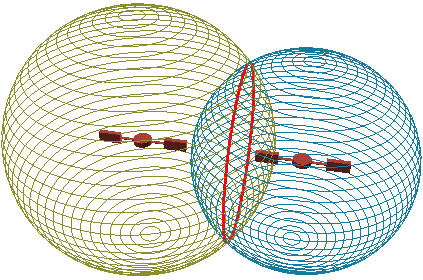Two satellite signals
This places us somewhere on the circle that is the intersection of the surface of the two spheres. This circle intersects with our true location on the surface of the earth, but given that we only know we are somewhere on the circle , we do not have enough information to limit the location to one point on the circle. This could give us a location somewhere on the surface of the earth, but our calculated location could also just as easily be located under the surface of the earth, or out in space.
Using the signal from a third satellite gives us more locational information. Intersecting a third sphere with the previous circle gives us two specific positions (marked with the black dot).
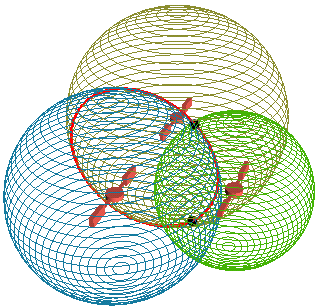Three satellite signals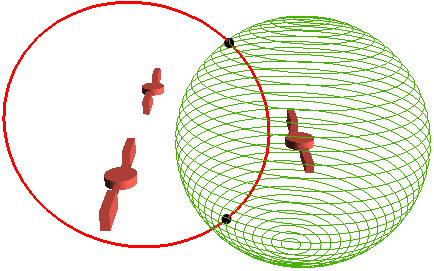 
One of these positions will be a precise location, and the other will be erroneous. We can therefore either throw out the location that is ridiculously far away (floating out in space, or under the surface of the earth).
Including a 4th satellite (not shown) also eliminates the impossible 2nd point, and gives the added benefit of more accurate locations.
Precise timing is so important, that an error of 1/1000 of a second would result in a positional error of about 300,000 m. If the clock within the receiver is running behind, the distances will be overestimated, and if the clock is running ahead, the distances will be underestimated.
As you can see, the GPS is dependent on precise timing between the satellite and the receiver. Each of the satellites contain atomic clocks, which are accurate to within a few hundred nanoseconds. These clocks cost about $100,000 apiece! Although the clocks in the GPS receivers are quite accurate, they are certainly not this accurate.
How, then, are the times synchronized? This is computed by the microprocessors within the receivers themselves. Because the microprocessor solves a series of simultaneous equations, these will only be able to be solved if the position is true. If no solution is found, the GPS microprocessor will add or subtract time until the solution to the simultaneous equations is found. The time differential is applied to the GPS receiver clock, which then becomes virtually as accurate as the atomic clocks in the satellites.
The satellites themselves are in predictable, continuous, non-geosynchronous orbit, far out in space. However, small variations in the earth's gravitational field, gravitation from the sun and moon, solar "wind," and other effects can perturb the satellites' orbit geometries or speeds.
The satellites are placed in very precise orbit, and the individual positions are monitored very closely by the DOD. Each satellite also contains its own GPS receiver, which allows it to know its own location.
Along with the time-stamped signals, each satellite also periodically broadcasts data regarding the satellite's position and health.
Although many potential sources of error exist, the overall error of GPS is quite low. With advanced receivers and differential correction, GPS locations can be brought to the sub-centimeter level of accuracy.
The base station records these time differences in files, which can be used to alter the times stored by the roving unit. When the roving unit's files are corrected, error can be reduced to 1-5 m error for standard resource-grade receivers.
Various government agencies maintain base stations and make their correction files available on the Internet.
Real-time DGPS
The US Coast Guard operates a series of DGPS base stations at various coastal positions in the USA. These base stations not only record correction files, but they also are equipped with AM radio broadcasting stations, which broadcast the correction values in real time. Other countries also operate broadcasting base stations.
For roving receivers that contain a second radio receiver, the correction factor can be applied immediately, which instantaneously brings the error down to a level suitable for many navigation and data collection needs.
Commercial services, some also using spaceborne satellites, offer real-time DGPS correction in non-coastal areas.
Most advanced receivers are shipped with software that will perform differential correction.
For example, the location of a series of utility poles can be recorded, along with the ID number, height, and condition of the pole. Forest stands can be outlined, along with attributes describing the number of trees per acre, dominant species, or burn status. Roads can be driven while the surface composition and condition, number of lanes, and route number are also recorded.
Import into the GIS
For GIS users, the last step is getting the data into a GIS. Most GPS vendors sell software that will export GPS data into various different GIS formats, such as the ArcGIS shapefile and ArcInfo's generate file format. Usually exporting is as easy as setting up a few menu choices and clicking a few buttons.
|
|||||||||||||||
|
The University of Washington Spatial Technology, GIS, and Remote Sensing Page is supported by the School of Forest Resources |
School of Forest Resources |
Syllabus