TAPESTRY: The Art of Representation and Abstraction
Derivative Geometry: Sweep along a line
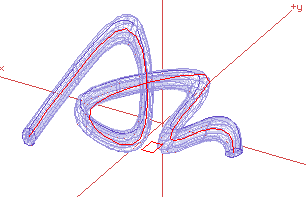SWEEPS, while conceptually similar to extrusions, involve a (potentially) much more complicated path. A simple sweep, using a simple path, might be indistinguishable from an extrusion, but a sweep such as the form•Z sweep shown at right can be quite complex. The red rectangle is going to be swept along the splined path to form a complex meshed object using Nurbs.
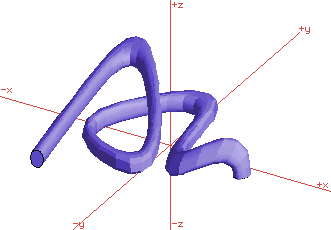The resulting form is generated by moving the cross-section in steps along the path, each time perpendicular to the local path segment, and connecting it with the polygons already generated.
Variations
As shown in the illustration, the basic framework of the sweep, which might involve a shape as simple as a rectangle, can be used to create quite complex shapes, such as the tube resulting from the sweep shown here.
"rails"
Some applications (incl Rhino) refer to the curve that defines the path as a "rail" and would call the sweep shown here as a sweep with one rail. A sweep with two rails uses the distance between the guide lines to define scale and orientation of the cross section during the sweep. A circle swept along two diverging lines can be made to define a cone, or horn shape.
end-caps
As with most of the "extrusion" operations, swept shapes form a tube which may optionally be "capped" or terminated with a closing polygon (making a solid). Alternatively, the end-cap might be rounded.
Mitred Corners
As with "wide p-lines" in Autocad and wide Postscript lines in Adobe Illustrator, geometry swept through acute corner angles can produce undesireable extensions of the corner. Controls for this might turn the corner in more than one step, keeping the extensions to a minimum.
Last updated: April, 2014
