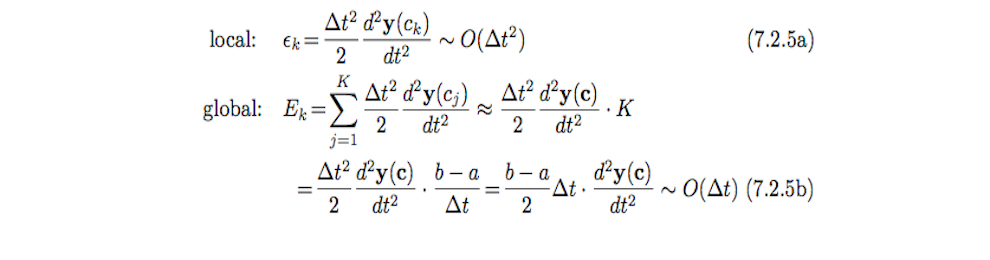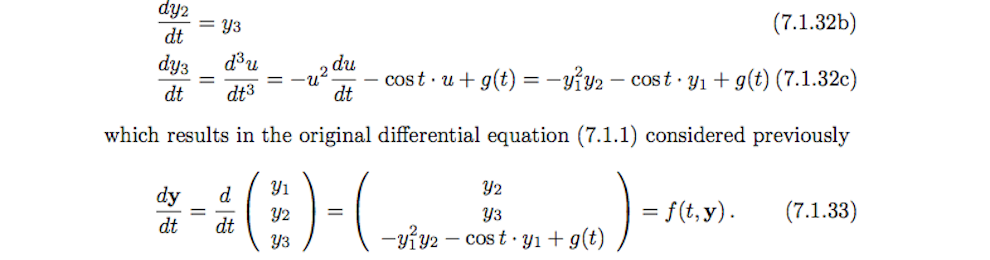Algorithms for Approximating Integral and Differential Calculus
LOADING
-
Time-Stepping Schemes for Future State Prediction
-
Local and Global Error in Time-Stepping
-
Stability Regions for the Euler Numerical Scheme
-
Systems of Differential Equations for Time-Stepping
-
Dynamical Systems that Exhibit Chaotic Behavior, i.e. the 3-body Problem
-
Two-Dimensional Quadrature for Integration
-
Cummulative Integration Rules
About Differential Calculus and Ordinary Differential Equations
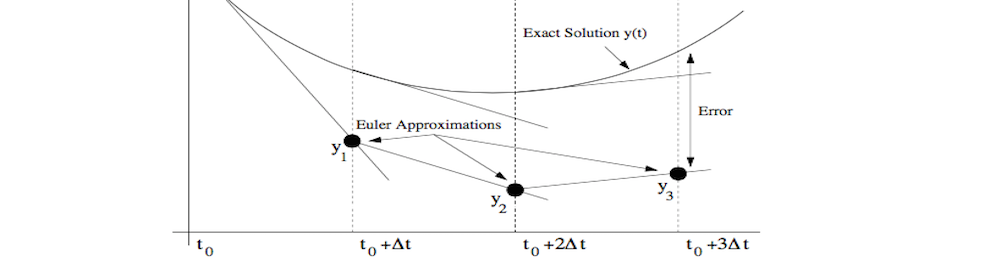
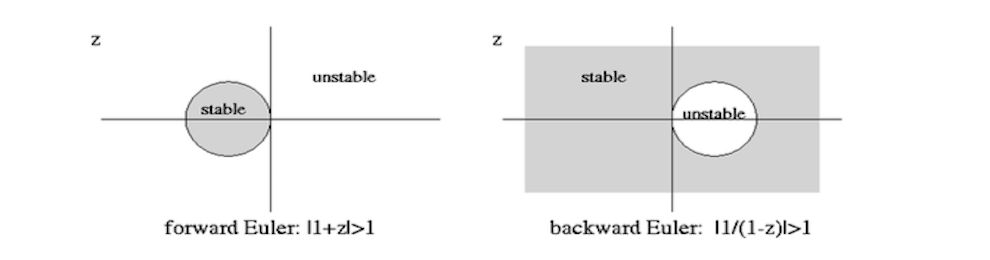
The development of numerical solution techniques for initial and boundary value problems originates from the simple concept of the Taylor expansion. Thus the building blocks for scientific computing are rooted in concepts from freshman calculus. Implementation, however, often requires ingenuity, insight, and clever application of the basic principles. In some sense, our numerical solution techniques reverse our understanding of calculus. Whereas calculus teaches us to take a limit in order to define a derivative or integral, in numerical computations we take the derivative or integral of the governing equation and go backwards to define it as the difference.